To calculate the value fo the account, we'll use the compund interest formula:
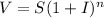
Where:
• V , is the final value of the account
,
• S ,is the amount saved from the begining
,
• I ,is the interest rate
,
• n ,is the number of times the interest is compounded
Now, we know that from graduation to one year after sophomore means a 3 years time frame. If the interest compounds every 6 months (semianually), it means that the interest was compounded 6 times.
Using this with the data given and the formula, we get that:
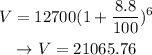
Thereby, the value of the account would be $21,065.76