Question

Calculate the value of the 1st term for the geometric sequence

Answer 1

we know taht

the explicit formula for an arithmetic sequence is equal to

`a_n=a_1+d(n-1)`

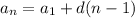
In this problem we have that

`a_n=-7+6n`

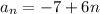
we know that

the distance betwee consecutive numbers is 6

that means

the common difference is d=6

substitute the value of d in the first equation

`\begin{gathered} a_n=a_1+6(n-1) \\ a_n=a_1+6n-6 \end{gathered}`

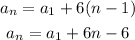
Equete both equations