Solution
We have the proportion of the freshwater fish is
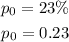
Now, from the sample we have the proportion
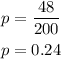
To test the hypothesis, we have

We have
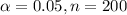
The test statistics
![z=\frac{p-p_0}{\sqrt[]{(p_0(1-p_0))/(n)}}](https://img.qammunity.org/2023/formulas/mathematics/college/kgg2m95d7p2pakqsfgeh95f4oc6ubhrnut.png)
Substituting the parameters, we obtain
![\begin{gathered} z=\frac{p-p_0}{\sqrt[]{(p_0(1-p_0))/(n)}} \\ z=\frac{0.24-0.23}{\sqrt[]{(0.23(1-0.23))/(200)}} \\ z=\frac{0.01}{\sqrt[]{(0.23*0.77)/(200)}} \\ z=0.3360514064 \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/hf63k0tkqpozymggs2w6irw0g7ym4ksqsv.png)
Therefore, the test statistics is
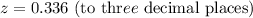
To find the P - value
Using the table, the p - value for the z score of 0.336 is

Notice that
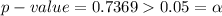
Therefore, since the p -value is greater than the level of significance, we fail to reject the null hypothesis, That is we support the null hypothesis