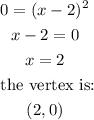Question

Determine the type and key parts of the graph of the function

Answer 1

Parabola

Vertex (2, 0)

Parabola opens: upward

Explanation:

We have the following function:

`y=\mleft(x-2\mright)^2`

Since x is squared, the form of the function is parabolic and having a plus sign in the dependent term, the parabola opens upwards.

The vertex would be when y = 0, since no parameter affects the function, therefore:

`\begin{gathered} 0=(x-2)^2 \\ x-2=0 \\ x=2 \\ \text{ the vertex is:} \\ (2,0) \end{gathered}`