A dilation is a type of transformation that changes the size of a figure by multiplying the coordinates of each vertex of the figure by a determined scale factor "k"
If the scale factor is greater than 1, then the dilation is an enlargement, i.e. the new figure is bigger.
If the scale factor is less than 1, then the dilation is a reduction, i.e. the new figure is smaller.
The general rule for dilation is:
Original → Dilation
(x,y) → (kx,ky)
As mentioned before you have to multiply each coordinate by the scale factor k=1/2
1/2 is less than 1, so we expect the figure to be smaller than the original.

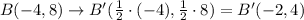
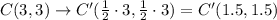
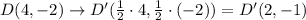
The resulting image will have the coordinates A'(0,2); B'(-2,4); C'(1.5,1.5); D'(2,-1) and it is a reduction.
The correct option is the third one.