Question

Find the length of the shorter leg in a 30 - 60 - 90 triangle where the hypotenuse is 10 units.

Answer 1

Hello!

First, let's draw this right triangle to understand better the problem:

Analyzing the draw, we can use the Property of Sine:

`\sin (30\degree)=\frac{\text{ opposite}}{\text{ hypotenuse}}`

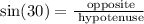
Remember that sin(30º) = 1/2.

Let's replace the values in the formula and solve it:

`(1)/(2)=\frac{\text{ x}}{\text{ 1}0}`

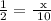
Multiplying across:

`\begin{gathered} (2\cdot x)=(1\cdot10) \\ 2x=10 \\ x=(10)/(2) \\ x=5 \end{gathered}`

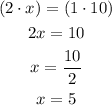
Right answer: D. 5 units.