Question

Solve the inequality both algebraically and graphically. Draw a number line graph of the solution and give interval notation.4x+3<−5x4+

Answer 1

The inequality given is

`4x+3<-(5x)/(4)+5`

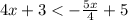
We take all the variables to one side and numbers to another. Then we solve it. The steps are shown below:

`\begin{gathered} 4x+3<-(5x)/(4)+5 \\ 4x+(5x)/(4)<5-3 \\ (21)/(4)x<2 \\ x<(2)/((21)/(4)) \\ x<2*(4)/(21) \\ x<(8)/(21) \end{gathered}`

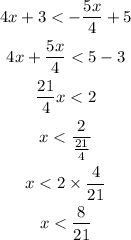
Solution

`x<(8)/(21)`

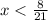
Now, in interval notation, we can write,

`(-\infty,(8)/(21))`

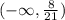
The graph of the solution in a number line would be all numbers LESS THAN 8/21.

Choice E shows this correctly.

Remember, in graph, "(" or ")" shows a number that isn't included.

"[" or "]" shows a number that is included.

Answer

------>>> Solution

`x<(8)/(21)`

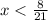
------>>> Solution (in interval notation)

`(-\infty,(8)/(21))`

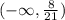
------>>> Solution (Graph)

E