Question

For each value below, enter the number correct to four decimal places.

Answer 1

We need to find the average velocity at each time interval.

The average velocity ΔV is the change in position at two moments divided by the change of time:

`\begin{gathered} \Delta V=(h_2-h_1)/(t_2-t_1)=(56t_2-0.83t_2^(2)-56t_1+0.83t_1^(2))/(t_2-t_1) \\ \\ \Delta V=(56(t_2-t_1)-0.83(t^2_2-t^2_1))/(t_2-t_1) \end{gathered}`

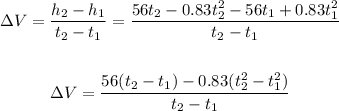
Using the above formula for each interval, we find:

• [7,8]:

`\Delta V=(56(8-7)-0.83(8^(2)-7^(2)))/(8-7)=56-0.83(15)=43.55`

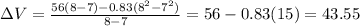
• [7,7.5]:

`\Delta V=(56(7.5-7)-0.83(7.5^2-7^2))/(7.5-7)=(56\mleft(0.5\mright)-0.83\mleft(7.25\mright))/(0.5)=43.965`

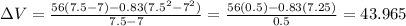
• [7,7.1]:

`\Delta V=(56(7.1-7)-0.83(7.1^2-7^2))/(7.1-7)=(56(0.1)-0.83(1.41))/(0.1)\cong44.2970`

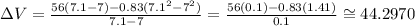
• [7,7.01]:

`\Delta V=(56(7.01-7)-0.83(7.01^2-7^2))/(7.01-7)=(56(0.01)-0.83(0.1401))/(0.01)=44.3717`

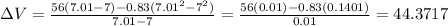
• [7,7.001]:

`\Delta V=(56(7.001-7)-0.83(7.001^2-7^2))/(7.001-7)=(56(0.001)-0.83(0.014001))/(0.001)\cong44.3792`

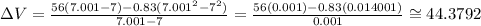
Therefore, the average velocities are:

[7,8]: 43.55 m/s

[7,7.5]: 43.965 m/s

[7,7.1]: 44.2970 m/s

[7,7.01]: 44.3717 m/s

[7,7.001]: 44.3792 m/s