Question

I would like help on this can you please help

Answer 1

Given:

A function

`f(x)=-2x^2+9`

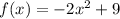
and value of g(x) at x.

To find:

Maximum value of both functions.

Step-by-step explanation:

For criticle points find f'(x) = 0 and f''(x)>0. Then value will be maximum.

Solution:

Now, first derivative is

`\begin{gathered} f^(x)=-2x^2+9 \\ f^(\prime)(x)=-4x \end{gathered}`

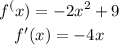
Now, put f'(x)=0 and criticle point will be 0.

Now,

As second derivative of function is negetive at x=0. So, we will get maximum at x=0

So, At x=0

`f(0)=9`

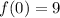
So, maximum value of f(x) is 9 and maximum value of g(x) is 11.

Hence, this is the maximum values of f(x) and g(x).