1) Let's sketch that to better understand.
2) Since tangent lines on the circle make right angles, and due to that bisector, we can state that we have two right triangles. So let's find out the measure of the radius.
We can find that by calculating the cosine of 68º
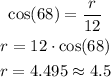
3) Hence, the measure of the radius is 4.5 units rounded off to the nearest tenth.