Question

Below is the graph of y = |x| . .Translate it to make it the graph of of y=|x-2|+4

Answer 1

Step-by-step explanation

Given a function f(x) we translate the function:

• a units horizontally (a > 0 to the right, a < 0 to the left),

,

• b units vertically (b > 0 up, b < 0 down),

by the transformation:

`f(x)\rightarrow g(x)=f(x-a)+b.`

In this case, we have:

`\begin{gathered} f(x)=|x|, \\ g(x)=|x-2|+4=f(x-2)+4. \end{gathered}`

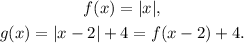
Comparing f(x) and g(x) with the general transformation above, we see that the graph of g(x) is the graph of f(x) translated:

• a = 2 units to the right,

,

• b = 4 units up.

Translating the graph of f(x), we get:

Answer

The translated graph is the graph in red: