So,
We're going to find the equation of the line that passes through the points J and K. To do this, we need to find the slope of the line first:

What we did, was to substract the y-coordinates of each point, and then divide this result by the substraction of the x-coordinates of each point. Therefore, the slope is 0.5.
Now, we could use the fact that the main equation of a line is given by the form:

Where m is the slope, b is the y-intercept, and (x,y) is a point.
We could replace the point K(6,5) and the slope 0.5 to find the value of b:
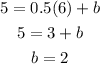
Therefore, the equation of the line given is:
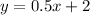
C. Now, if there's a new line LM, the system of linear functions made could have three possible solutions:
1. It could happen that both lines are parallel (They have the same slope) with a different "b" intercept so they will never intersect. If this happens, the system has not solution.
2. It could happen that both lines intersect at only one point. That means that the system has only one solution.
3. It could happen that both lines are the same line. So the system could have infinite solutions.