Question

Antonio deposits $1,750 into an account that earns 5% interest compounded daily. How much interest does he earn after 1 day?

Answer 1

He earns $0.24 in interest after 1 day.

Explanation:

Compound interest:

`A(t)=P(1+(r)/(n))^(n\ast t)`

In which:

P is the principal, the amount of the initial deposit.

r is the yearly interest rate, as a decimal.

n is the number of compoundings during a year.

t is the number of years.

In this question:

Deposit of $1,750, so P = 1750.

Interest rate of 5%, so r = 0.05.

Compounded daily. A year has 365 days, so n = 365.

After 1 day. 1 day is 1/365 of an year, so t = 1/365.

The amount he will have after one day will be:

`A=1750(1+(0.05)/(365))^{(1)/(365)\ast365}=1750(1+(0.05)/(365))=1750.24`

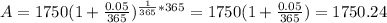
The interest earned will be:

Amount after 1 day subtracted by the deposit.

Deposit of $1,750.

After 1 day, he will have $1,750.24.

1750.24 - 1750 = 0.24

He earns $0.24 in interest after 1 day.