x=Let's illustrate the problem first. Assuming the height of the slide is right-angled to the ground.
To determine the length of the slide, we can use sine function.
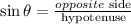
In the illustration, the opposite side of the angle 45° is 20ft whereas the hypotenuse is the length of the slide. Let's plug in these values to the function above. Assume x = length of the slide.

Solve for x.
![\begin{gathered} x=(20ft)/(\sin 45) \\ x=20\sqrt[]{2}ft \\ x\approx28.28ft \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/3b35658xe1zw908bdkx9en9u1gamkkf9io.png)
The length of the slide and the distance the child has to travel down the slide is approximately 28.28 ft. (Option C)