Question

Give the perpendicular slope of the given line y=8/3x-4

Answer 1

To calculate the perpendicular slope, the steps are the following:

• Step 1: Find the original slope of the line

,

• Step 2: Use the condition for the perpendicular slope, to find the perpendicular slope.

Step 1. To find the slope of the given line

`y=(8)/(3)x-4`

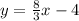
we need to compare this with the slope-intercept equation

`y=mx+b`

where m is the slope and b is the y-intercept of the line. Thus, we can see that the number that accompanies the x represents the slope. And in this line

`y=(8)/(3)x-4`

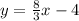
That number is 8/3. I will call this the slope m1:

`m_1=(8)/(3)`

Step 2. The condition for two lines m1 and m2 to be perpendicular is:

`m_1* m_2=-1`

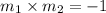
Since in this case we know the original slope m1, and we are looking for perpendicular slope m2, we substitute m1, and solve for m2:

`(8)/(3)* m_2=-1`

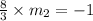
Solving for m2, we need to multiply each side of the equation by 3/8:

`(3)/(8)*(8)/(3)* m_2=-1*(3)/(8)`

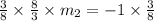
On the left side we are only left with m2, and on the right side we are left with -3/8:

`m_2=-(3)/(8)`

Answer: -3/8