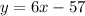Question

Find the slope intercept form of the equation parallel to the line of Y =6x+72 and with coordinates of (8-9).Then find the slope intercept form of the equation Perpendicular to the line Y=5x-1/2 and with the coordinates of (25,-11).

Answer 1

`y=6x-57`

Step-by-step explanation

the slope -intercept form of the equation of a line is

`\begin{gathered} y=mx+b \\ where\text{ m is the slope} \\ b\text{ is the y-intercept} \end{gathered}`

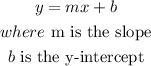
so

Step 1

find the slope of the line:

2 lines that are parellel has the same slope , so the slope of the line we are looking for must equal to the slope of

`\begin{gathered} y=6x+7 \\ y=6x+7\Rightarrow y=mx+b \\ so \\ m=slope=6 \end{gathered}`

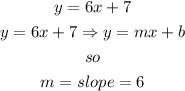
so

slope=6

Step 2

now, we need to use the point-slope formula , it says

`\begin{gathered} y-y_1=m(x-x_1) \\ where \\ m\text{ is the slope and } \\ P1(x_1,y_1)\text{ is a well known of the line} \end{gathered}`

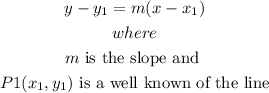
so

a) let

`\begin{gathered} slope=6 \\ P1(8,-9) \end{gathered}`

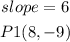
b) now, replace and solve for y

`\begin{gathered} y-y_(1)=m(x-x_(1)) \\ y-(-9)=6(x-8) \\ y+9=6x-48 \\ subtract\text{ 9 in both sides} \\ y+9-9=6x-48-9 \\ y=6x-57 \end{gathered}`

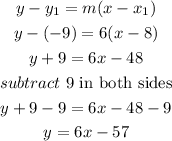
therefore, the answer is

`y=6x-57`

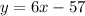
I hope this helps you