Question

Write the equation's of the lines using the given information m=1/4, (4, 3)

Answer 1

Given the question in the question tab, the following are the steps to solve the problems.

Step 1: Write the general equation of a line

`\begin{gathered} y=mx+c \\ \text{where m=slope, c=y-intercept} \end{gathered}`

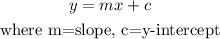
Step 2: Write the given parameters

`m=(1)/(4),(x,y)=(4,3),c=\text{?}`

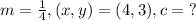
Step 3: Calculate the y-intercept (c) by substituting the values into the equation in step 1

`\begin{gathered} y=mx+c \\ 3=(1)/(4)(4)+c \\ 3=1+c \\ 3-1+c \\ c=2 \end{gathered}`

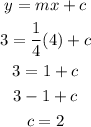
Step 4: Write the equation of the line

`\begin{gathered} y=mx+c \\ y=(1)/(4)(x)+2 \\ y=(x)/(4)+2 \end{gathered}`

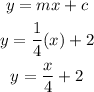
Hence, the equation of the line is:

`y=(x)/(4)+2`