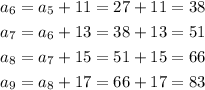Question

Sandra wrote the sequence shown.3, 6, 11, 18, 27, ...What is the 9th term of the sequence?Ο Α. 48OB. 58O C. 83O D. 102

Answer 1

We are given the first five elements of the sequence.

Let's call them as follows:

`\begin{gathered} a_1=3 \\ a_2=6 \\ a_3=11 \\ a_4=18 \\ a_5=27 \end{gathered}`

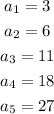
Now, we want to figure out what is the difference between consecutive elements from the sequence.

We have:

`\begin{gathered} a_2-a_1=6-3=3 \\ a_3-a_2=11-6=5 \\ a_4-a_3=18-11=7 \\ a_5-a_4=27-18=9 \end{gathered}`

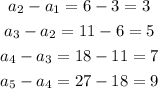
So, to find an element we are not adding a constant value (like in arithmetic sequences) nor we are multiplying by a constant (like in geometric sequences). Nevertheless we can see that the sequence of the differences between consecutive elements is equal to the odd numbers (3, 5, 7, 9).

So, we just have to keep writting the sequence, adding the next odd number:

`\begin{gathered} a_6=a_5+11=27+11=38 \\ a_7=a_6+13=38+13=51 \\ a_8=a_7+15=51+15=66 \\ a_9=a_8+17=66+17=83 \end{gathered}`