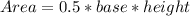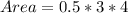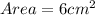Answer:
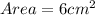
Explanation:
Given
See attachment for polygons
Required
The area of the other polygon
From the attachment, we have:
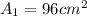
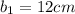
and

In
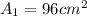 and
and
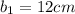
The area is calculated as:
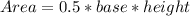
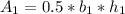
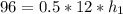

Solve for h
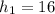
Next, is to calculate the height of the other polygon using the following equivalent ratios;
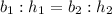
This gives:
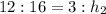
As fraction
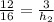
Solve for h
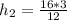
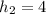
So, the area is