Question

A 3 kg hard steel ball collides head-on with a 1 kg hard steel ball. The balls are moving up at 2 m/s in opposite directions before they collide. Upon colliding, the 3 kg ball stops. What is the velocity of the 1 kg object after the collision?

Answer 1

We know that the momentum is conserved this means that:

`p_(1i)+p_(2i)=p_(1f)+p_(2f)`

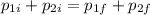
where

`p=mv`

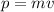
In this case the balls are moving opposite each other this means that the velocities have opposite sign, we also know that the 3 kg ball stops after the collision this means that it final velocity is zero, then we have:

`\begin{gathered} (3)(2)-(1)(2)=(3)(0)+1v_(2f) \\ 6-2=v_(2f) \\ v_(2f)=4 \end{gathered}`

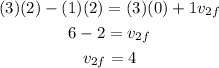
Therefore the velocity of the 1 kg ball after the collision is 4 m/s