Question

I need help with number 2 to make sure I’m doing it correctly

Answer 1

Question 2.

Given the system of equations:

• -x + y = 1

,

• x + y = 11

Let's solve the system by elimination method using addition and subtraction.

To solve the system, add both equations to eliminate the x variable.

-x + y = 1

+ x + y = 11

__________

2y = 12

Divide both sides of the equation by 2:

`\begin{gathered} (2y)/(2)=(12)/(2) \\ \\ y=6 \end{gathered}`

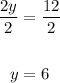
Now, substitute 6 for y in either of the equations:

Let's take the second equation

`\begin{gathered} x+y=11 \\ \\ x+6=11 \\ \\ \text{Subtract 6 from both sides:} \\ x+6-6=11-6 \\ \\ x=5 \end{gathered}`

Therefore, we have the solution:

x = 5, y = 6