Question

A circle with a center of (-4,5) passes through the point (2,3). What is its equation in standard form?

Answer 1

The correct option is C.

`(x+4)^2+(y-5)^2=40`

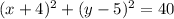
THe standard form of a circle is :

`\mleft(x-a\mright)^2+(y-b)^2=r^2`

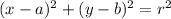
Where (a, b) is the coordinates of the center and r is the radius,

We know the center, we need to find the radius. The radius is equal to the distance to any point of the circle to the center of the circle. Having 2 points P = (c, d) and Q = (e, f) the distance is:

`d=\sqrt[]{(c-e)^2+(d-f)^2}`

THen, in this case we have the points (-4, 5) and (2, 3). To find hte radius, w