Question

Write a liner equation to represent the line shown on the graph

Answer 1

The slope of a line that passes through the points (x1, y1) and (x2, y2) is computed as follows:

`m=(y_2-y_1)/(x_2-x_1)`

The point-slope form of a line is:

`y-y_1=m(x-x_1_{})`

7) The line passes through (0,-2) and (3,2)

Slope:

`m=(2-(-2))/(3-0)=(4)/(3)`

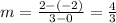
Equation:

y - 2 = 4/3(x - 3)

8) The line passes through (0,5) and (4,3)

Slope:

`m=(3-5)/(4-0)=-(2)/(4)=-(1)/(2)`

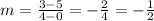
Equation:

y - 3 = -1/2(x - 4)

9) this line is parallel to the y-axis, then it has the form:

x = constant

In this case, the equation is:

x = 1