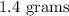The amount of a decaying substance with half life τ that remains after a time t, if the initial amount of that substance is A_0, is given by the formula:
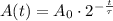
If the half life of that substance is 12 days, and the initial amount of that substance is 4.5 grams, then A_0=4.5 and τ=12. Substitute those values as well as t=20 to find the remaining amount after 20 days:
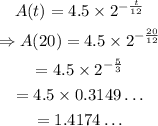
To the nearest tenth of a gram, the remaining amount after 20 days, is: