Question

1 poi (7) Write the equation of a line that has a slope of -5 and passes through the point (2, -13)

Answer 1

Explanation:

The equation of a line in slope-intercept form is

`y=mx+b`

where m is the slope and b is the y-intercept.

Now we know that the slope is -5; therefore m = -5 and our equation becomes

`y=-5x+b`

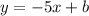
Now we only need to find the y-intercept b of the line, and to do this we use the point (2, -13). Putting in x = 2 and y = -13 in the above equation gives

`-13=-5(2)+b`
`-13=-10+b`

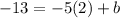
Adding 10 to both sides gives

`-13+10=b`
`b=-3`

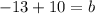
With the value of b in hand, we now write the equation in slope-intercept form

`y=-5x-3`

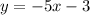
which is our answer!