First, let's check if all segments have the same length, calculating the distance between the points using the formula:
![d=\sqrt[]{(y_2-y_1)^2+(x_2-x_1)^2}](https://img.qammunity.org/2023/formulas/mathematics/college/nkjymhkzx142t3t66rnvx6qo7qj0ya3b8k.png)
So we have:
![\begin{gathered} AB\colon \\ d=\sqrt[]{(-1-4)^2+(4-2)^2}=\sqrt[]{25+4}=\sqrt[]{29} \\ \\ BC\colon \\ d=\sqrt[]{(-3-(-1))^2+(-1-4)^2}=\sqrt[]{4+25}=\sqrt[]{29} \\ \\ CD\colon \\ d=\sqrt[]{(2-(-3))^2+(-3-(-1))^2_{}_{}}=\sqrt[]{25+4}=\sqrt[]{29} \\ \\ AD\colon \\ d=\sqrt[]{(4-2)^2+(-3-2)^2}=\sqrt[]{4+25_{}}=\sqrt[]{29} \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/kk2v6uw2yjcir72vyuo1z3ah173x4vy0kb.png)
Now, we need to check the slopes of each segment. The adjacent sides need to be perpendicular, so their slopes need to have the relation:
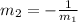
Calculating the slopes with the formula below, we have:
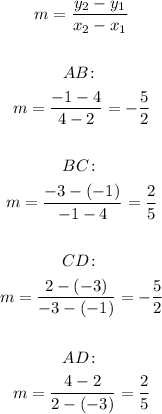
So all adjacent sides are perpendicular.
All sides have the same length and all adjacent sides are perpendicular, therefore ABCD is a square.