Question

At which values of x does the graph of the function F(x) have a vertical asymptote? Check all that apply.A.x = -3B.x = 8C.x = 3D.x = -8E.x = 4F.x = -4

Answer 1

Given the function:

`F(x)=(x+4)/(x^2+5x-24)`

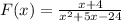
Let's find the values of x where the graph of the function has a vertical asymptote.

Let's factor the denominator using the AC method.

Find a pair of numbers whose sum is 5 and whose product is -24.

We have:

8 and -3

Thus, the function will be:

`F(x)=(x+4)/((x+8)(x-3))`

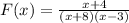
The vertical asymptote(s) are the values of x where the function is undefined.

Thus, they are the values where the denominator will tend to zero.

Write each factor in the denominator to zero and solve for x.

We have:

• x + 8 = 0

Subtract 8 from both sides:

x + 8 - 8 = 0 - 8

x = -8

• x - 3 = 0

Add 3 to both sides:

x - 3 + 3 = 0 + 3

x = 3

Therefore, the values of x where the function have a vertical asymptote is:

x = -8 and x = 3

ANSWER:

• C. x = 3

• D. x = -8