Question

Suppose the expression ab)" models the approximate number of customers who applied for a credit card every month since a bank opened,where a is the initial number of customers who applied, bis the rate of increase in the number of people who applied every month, and n is thenumber of months since the bank opened.if the expression below models the number of applicants for a credit card, what is the correct interpretation of the second factor?27(1.2)

Answer 1

From the statement of the problem, we know that the number of customers is modelled by the equation:

`N=a\cdot b^n.`

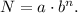
Where:

• a = initial number of customers,

,

• b = rate of increase in the number of people per month,

,

• n = number of months.

We have this particular equation:

`N=27\cdot(1.2)^4\text{.}`

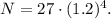
Comparing this equation with the general one above, we see that:

• a = 27,

,

• b = 1.2,

,

• c = 4.

Computing the value of the second factor, we have:

`N\cong27\cdot2.07.`

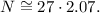
We see that after 4 months the number of customers will be 2.07 times the initial number of customers (27).

Answer

A. There were 2.07 times as many customers who applied after the 4th month compared to the initial number of customers.