Question

May I please get help with this? I’m confused as to how I can get the correct answer

Answer 1

25 ft

Step-by-step explanation

as the mirror is on the ground totally hoziontal , the angle is the same for both triangles,hence, the triangles are congruent, so we can set a proportion

Step 1

set the proportion

let

`\text{ratio}=\frac{heigth}{\text{below side}}`

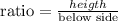
replace

`\begin{gathered} \text{ratio}_1=(5)/(7.7) \\ \text{ratio}_2=\frac{heigth\text{ of the tr}e(T)}{39} \end{gathered}`

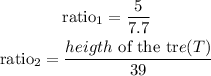
the triangles are congruent, so

`\begin{gathered} \text{ratio}_1=ratio_2 \\ (5)/(7.7)=(T)/(39)\rightarrow proportion \end{gathered}`

Step 2

solve the equation

`\begin{gathered} (5)/(7.7)=(T)/(39) \\ \text{Multiply both sides by 39} \\ (5)/(7.7)\cdot39=(T)/(39)\cdot39 \\ 25.32=T \\ \text{rounded} \\ T=25\text{ ft} \end{gathered}`

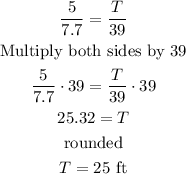
so, the answer is

25 ft

I hope this helps you