Question

Emily and Adam are selling pies for a school fundraiser. Custoemrs can buy blueberry pies and pumpkin pies. Emily sold 12 blueberry pies and 13 pumpkin pies for a total of $278. Adam sold 11 blueberry pies and one pumpkin pie for a total of $102. What is the cost of each of one blueberry pie and one pumpkin pie?

Answer 1

Emily: 12 blueberry

13 pumpkin

total: $278

Adam: 11 blueberry

1 pumpkin

total: $102

This is a system of 2 equations with 2 variables. Let's call:

price of blueberry pie: x

price of pumpkin pie: y

The equations are:

`\begin{gathered} 12x+13y=278 \\ 11x+y=102 \end{gathered}`

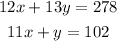
So we have to solve this system. I'll do it by clearing y in the second equation and replacing in into the first equation:

`\begin{gathered} 11x+y=102 \\ y=102-11x \end{gathered}`

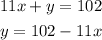
And we replace into the first equation:

`\begin{gathered} 12x+13y=278 \\ 12x+13(102-11x)=278 \end{gathered}`

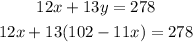
And clear this one for x:

`\begin{gathered} 12x+13\cdot102-13\cdot11x=278 \\ 12x-143x=278-1326 \\ -131x=-1048 \\ x=(-1048)/(-131)=8 \end{gathered}`

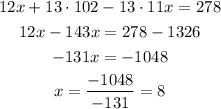
If x = 8 it means that the price of the blueberry pie is $8

Then, to find y we just have to replace this value of x into the equation we cleared for y before:

`\begin{gathered} y=102-11x \\ y=102-11\cdot8 \\ y=102-88 \\ y=14 \end{gathered}`

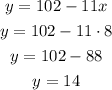
The price of the pumpkin pie is $14