Step 1; Identify the quantites
volume = V
Temperature = T
Pressure = P
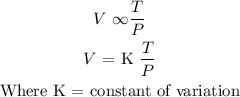
V = 645, T = 430°, P = 10
Step 2 : substitute the value above into the formula to find the value of K
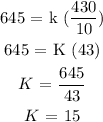
The relationship between the variables is given as
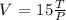
Step 3: Find the volume, when T = 100°, P = 20
Then substitute the values into the relationship to find V
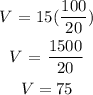
Therefore , volume V is 75