Question

Suppose that the height (in centimeters) of a candle is a linear function of the amount of time (in hours) it has been burning. After 6 hours of burning, a candlehas a height of 19.4 centimeters. After 20 hours of burning, its height is 11 centimeters. What is the height of the candle after 8 hours?

Answer 1

Given that the height of a candle (in centimeter) is a linear function of time (in hour) it has been burning.

Let at time t, the height of the candle be h

Since h is a linear function of t, let us assume

`h=at+b`

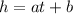
After 6 hours of burning, the candle has a height of 19.4 centimeters.

After 20 hours of burning, its height is 11 centimeters.

So, the line representing h passes through the points (6,19.4) and (20,11)

Using two-point formula

`\begin{gathered} (h-11)/(19.4-11)=(t-20)/(6-20) \\ (h-11)/(8.4)=(t-20)/(-14) \\ h=-(3)/(5)t+23 \end{gathered}`

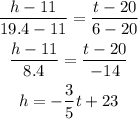
So, the height of the candle is

`h=-(3)/(5)t+23`

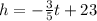
Now, putting t=8, it gives

`\begin{gathered} h=23-(3)/(5)*8 \\ =23-(24)/(5) \\ =(115-24)/(5) \\ =(91)/(5) \end{gathered}`

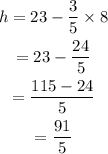
So, after 8 hours, the height of the candle is 18.2 centimeters.