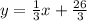Question

which of the following lines is perpendicular to the line Y=-3x+2 and passes through the point (-2,8)

Answer 1

Given the equation of the line:

y = 3x + 2

The perpendicular line passes through the point: (-2, 8)

Let's find the equatrion of the perpendicular line.

The slope of a perpendicular line is the negative reciprocal of the slope of the original line.

Apply the slope-intercept form:

y = mx + b

Where m is the slope and b is the y-intercept.

y = -3x + 2

The slope here is -3

Therefore, the slope of the perependicular line is the negative reciprocal of -3.

We have:

`\begin{gathered} m_1m_2=-1 \\ \\ m_2=-(1)/(m_1) \\ \\ m_2=-(1)/(-3) \\ \\ m_2=(1)/(3) \end{gathered}`

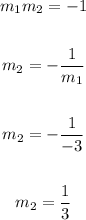
The slope of the perpendicular line is ⅓.

From the slope-intercept form, substitute ⅓ for m:

y = mx + b

`y=(1)/(3)x+b`

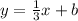
To find the value of the y-intercept, b, substitute the points (x, y) ==> (-2, 8) for x and y respectively:

`\begin{gathered} 8=(1)/(3)(-2)+b \\ \\ 8=-(2)/(3)+b \\ \\ \end{gathered}`

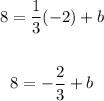
Multiply all terms by 3:

`\begin{gathered} 8(3)=-(2)/(3)(3)+3b \\ \\ 24=-2+3b \end{gathered}`

Add 2 to both sides:

`\begin{gathered} 24+2=-2+2+3b \\ \\ 26=3b \end{gathered}`

Divide both sides by 3:

`\begin{gathered} (26)/(3)=(3b)/(3) \\ \\ (26)/(3)=b \\ \\ b=(26)/(3) \end{gathered}`

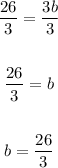
Therefore, the equation of the perpendicular line is:

`y=(1)/(3)x+(26)/(3)`

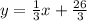
ANSWER:

`y=(1)/(3)x+(26)/(3)`