Question

A car is 35.3m down the road from the lizard driving toward it at a speed of 30.5 m/s. How long will it take the car to drive 35.3 m? Round to a tenth of a second.

Answer 1

We need to find the time that the car takes to travel 35.3m if it is being driven at 30.5m/s.

Let's remember how velocity is definded:

`V=(\Delta x)/(\Delta t)`

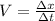
Where ∆x is the distance traveled, ∆t is the time taken to travel that distance, and V is the velocity. For this case we know velocity (V = 30.5 m/s) and the distance (∆x = 35.3 m). We need to find ∆t. Let´s replace values in the equation:

`30.5m/s=(35.3m)/(\Delta t)`

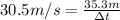
We just need to solbe for ∆t:

`\Delta t=(35.3m)/(30.5m/s)\approx1.1574s`

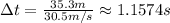
The car takes approximately 1.2 seconds (rounded to the nearest tenth of a second) to travel that distance.

Now, to know if the lizard will make it to the other side before the car gets there, we need to calculate the time the lizard takes to cross the road. We calculate it following the sae process we used for the car. For this case V = 2.8m/s and ∆x = 3 m. The lizard needs to cross the road in 1.2 seconds or less. Let's see:

`2.8m/s=(3m)/(\Delta t)`
`\Delta t=(3m)/(2.8m/s)\approx1.071s`

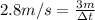
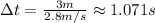
The lizard corsses the road in 1.1 seconds (rounded to the nearest tenth of a second). He takes less time than te car, hence, the lizard will be able to cross the road before the car gets there.