Given:
The heat energy added to the sample is Q = 130 J
The mass of each sample is m = 1 kg
The specific heat of aluminum is
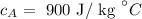
The specific heat of brass is
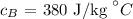
The specific heat of glass is
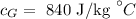
The specific heat of steel is

Required: The sample that would require the greatest temperature increase.
Step-by-step explanation:
The temperature increase for a sample can be calculated by the formula
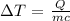
On substituting the values, the temperature increase of aluminum will be
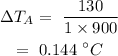
On substituting the values, the temperature increase of brass will be
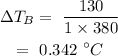
On substituting the values, the temperature increase of glass will be
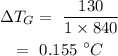
On substituting the values, the temperature increase of steel will be
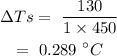
Final Answer: The greatest temperature increase is experienced by brass.