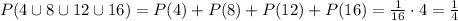Question

the carnival spinner shown is divided into equal sections . on every spin,each outcome is equally likelythe spinner lands on a number less than 10. What is the probability that the number is a multiple of four?

Answer 1

There are 16 possible outcomes on the carnival spinner, each one of them has the same probability, the probability can be calculated as the nº successes by the total number of outcomes:

`P(.)=\frac{1}{\text{total outcomes}}=(1)/(16)`

`P(1)=P(2)=P(3)=P(4)=P(5)=P(6)=P(7)=P(8)=P(9)=P(10)=P(11)=P(12)=P(13)=P(14)=P(15)=P(16)=(1)/(16)`

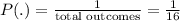
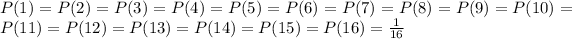
You have to calculate the probability that it lands on a number less than 10, you can symbolize this as

`P(X<10)`

The outcomes that meet the condition "being less than 10" are 1, 2, 3, 4, 5, 6, 7, 8, 9

So that

`P(X<10)=P(1)+P(2)+P(3)+P(4)+P(5)+P(6)+P(7)+P(8)+P(9)=9\cdot(1)/(16)=(9)/(16)`

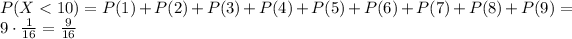
The probability of the spinner landing in a number multiple of 4, the possible outcomes that meet this condition are: 4, 8, 12 and, 16

So the calculation is

`P(4\cup8\cup12\cup16)=P(4)+P(8)+P(12)+P(16)=(1)/(16)\cdot4=(1)/(4)`