Answer:
(a)Approximately 99.7% of women over 70 have blood pressures between 104 mmHg and 158 mmHg.
(b)Approximately 68% of women over 70 have blood pressures between 122 mmHg and 140 mmHg.
Explanation:
Given:
• Mean = 131 mmHg
,
• Standard Deviation = 9 mmHg.
By the empirical rule, in a normal distribution:
• 68% of the data falls within one standard deviation.
,
• 95% percent within two standard deviations, and
,
• 99.7% within three standard deviations from the mean.
(a)
As given by the empirical rule above, 99.7% of data in a normal distribution falls within three standard deviations from the mean. That is:
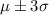
Substitute the given values:
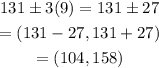
Approximately 99.7% of women over 70 have blood pressures between 104 mmHg and 158 mmHg.
(b)As given by the empirical rule above, 68% of data in a normal distribution falls within one standard deviation from the mean. That is:
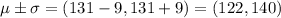
Approximately 68% of women over 70 have blood pressures between 122 mmHg and 140 mmHg.