Question

Write in the factored form by factoring out the greatest common factor (or negative common factor if the coefficient of the term of greatest degree is negative)

Answer 1

`-7x^6(6x+1)`

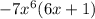
Step-by-step explanation

Step 1

Find the GCF of all the terms in the polynomial.

`\begin{gathered} -42x^7-7x^6 \\ \end{gathered}`
`\begin{gathered} -42x^7=-1\cdot2\cdot3\cdot7\cdot x^{}\cdot x\cdot x\cdot x\cdot x\cdot x\cdot x \\ -7x^6=-1\cdot7\cdot x\cdot x\cdot x\cdot x\cdot x\cdot x \end{gathered}`

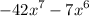
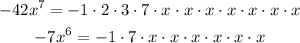
so ,

`\begin{gathered} \text{the GCF of }-42x^7-7x^6\text{ is} \\ -7x^6 \end{gathered}`

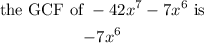
Step 2

Express each term as a product of the GCF and another factor.

`-42x^7-7x^6=(6x\cdot-7x^6)+(1\cdot-7x^6)`

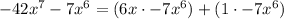
Step 3

Use the distributive property to factor out the GCF.

`\begin{gathered} (6x\cdot-7x^6)+(1\cdot-7x^6)=-7x^6(6x+1) \\ \end{gathered}`

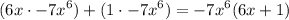
so, the answer is

`-7x^6(6x+1)`

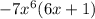
I hope this helps you