Question

4. You are on vacation sight-seeing at the coast and climb up to thetop of a 50-meter light house. You look down to see a boat andmeasure the angle of declination to be 17.5 degrees. How far away isthe boat from the base of the lighthouse?

Answer 1

First lets make a diagram of the situation

The lighthouse and the boat form a right triangle. The height of the light house is the adjascent side to the angle of declination.

You need to calculate the distance of the boat to the base of the light house, in the triangle, this is the side oposite to the angle of declination

To calculate the measure of the opposite side you have to use the trigonometric relations. In this case the trigonometric function that relates the adjacent and opposite sides is the tangent

`\tan \theta=(opposite)/(adjacent)`

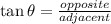
For θ=17.5 and adjacent side 50m

`\begin{gathered} \tan \theta\cdot adjacent=opposite \\ \text{opposite}=\tan 17.5\cdot50 \\ \text{opposite}=15.76 \end{gathered}`

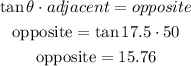
THe distance of the boat from the base of the light house is 15.76m