Question

A final exam in Math 160 has a mean of 70 with standard deviation 8. If 16 students arerandomly selected, find the probability that the mean of their test scores is less than 77.(hint: Central limit theorem)

Answer 1

Given:

`\begin{gathered} \mu=70 \\ \sigma=8 \\ n=16 \\ x=77 \end{gathered}`

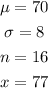
Using the Z-score formula;

`Z=(x-\mu)/((\sigma)/(√(n)))`

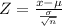
Substituting the values to get the Z-score,

`\begin{gathered} Z=(77-70)/((8)/(√(16))) \\ Z=(7)/((8)/(4)) \\ Z=(7)/(2) \\ Z=3.5 \end{gathered}`

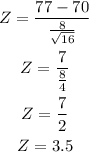
The probability that the mean of the test scores is less than 77 is gotten from Z-score tables.

From the Z-score table,

[tex]P(x

Therefore, the probability that the mean of the test scores is less than 77 is approximately 0.9998