Question

How can I find the min/max velocity with a 100g weight on a spring? And what would you predict the kinetic energy to be (min/max)

Answer 1

`\begin{gathered} v_(\max )=\sqrt[]{25* k\Delta x^2} \\ \Delta x^2=\text{ Max Stretch} \end{gathered}`

Explanation:

We need to find the max and min velocity conditions. In the spring-mass system harmonic oscillator, we have the following conditions.

(1) Maximum stretched

At a maximum stretch, kinetic energy is 0 therefore 0 velocity and potential energy is maximum

`\begin{gathered} KE=(1)/(2)mv^2=0 \\ PE=(1)/(2)k\Delta x^2=\text{Max} \\ \therefore\Rightarrow \\ ME=KE+PE\Rightarrow(1)/(2)mv^2+(1)/(2)k\Delta x^2 \\ ME=0+(1)/(2)k\Delta x^2 \\ ME=(1)/(2)k\Delta x^2 \\ \end{gathered}`

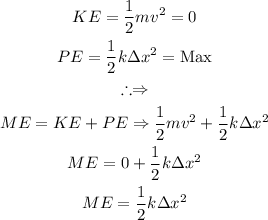
Note! that maximum stretch can be negative and positive, depending on the direction.

(2) Equlibrium position/ no stretch

`\begin{gathered} KE=(1)/(2)mv^2=\text{Max} \\ PE=(1)/(2)k\Delta x^2=0 \\ \therefore\Rightarrow \\ M.E=KE+PE\Rightarrow(1)/(2)mv^2+(1)/(2)k\Delta x^2 \\ M.E=(1)/(2)mv^2+0 \\ \text{Therefore, the Max/Min velocity can be found using these two conditions (1) and (2)} \\ v_(\max )=\sqrt[]{(2* M.E)/(m)} \\ v_(\max )=\sqrt[]{(2* M.E)/(100)}=\sqrt[]{50* M.E} \\ \text{SInce Mechanical energy is constant, therefore} \\ v_(\max )=\sqrt[]{25* k\Delta x^2} \\ \text{Where: } \\ \Delta x=\text{ Max Stretch} \\ \\ \\ \end{gathered}`