Question

A pole that is 3.5 m tall casts a shadow that is 1.55 m long. At the same time, a nearby building casts a shadow that is 45.25 m long. How tall is the building? Round your answer to the nearest meter.

Answer 1

We can solve this problem with proportions, by expressing the ratio of the height of the pole and the building to the length of their shadow, like this:

Height of pole / shadow of pole = Height of building / shadow building

when we take x as the height of the building and substitute the values provided by the question, we get:

`(3.5)/(1.55)=(x)/(45.25)`

From this expression we can solve for x, like this:

`\begin{gathered} (3.5)/(1.55)=(x)/(45.25) \\ (3.5)/(1.55)*45.25=(x)/(45.25)*45.25 \\ (3.5)/(1.55)*45.25=(45.25)/(45.25)* x \\ (3.5)/(1.55)*45.25=1* x \\ (3.5)/(1.55)*45.25=x \\ x=(3.5)/(1.55)*45.25\approx102 \end{gathered}`

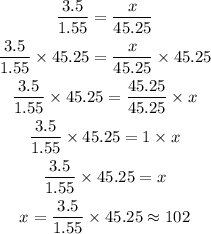
Then, the height of the building is 102 m