Question

A proportional relationship is represented by the equation y = 3x. Identify the constant of proportionality, make a table, and sketch a graph of the relationship. Identify the constant of proportionality.

Answer 1

a) Proportional relationships have the following form:

`y=kx`

Where "k" is the Constant of proportionality.

For this case you have this equation that represents a Proportional relationship:

`y=3x`

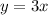
So you can identify that the Constant of proportionality is:

`k=3`

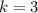
b) To make a table you must use different x-values, substitute them into the equation and evaluate, in order to get their corresponding y-values.

- For:

`x=-2`

You get:

`y=3(-2)=-6`

- For:

`x=-1_{}`

You get that:

`y=3(-1)=-3`

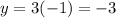
- For:

`x=2`
`y=3(2)=6`

- For:

`x=4`
`y=3(4)=12`

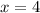
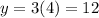
Knowing those coordinates, you can make the following table:

c) Use the table the sketch the graph of the relationship.

You can see in the table that you have the following points:

`(-2,-6),(-1,-3),(2,6),(4,12)`

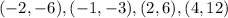
Plot these points on a Coordinate plane. The line must pass through those points (It's important to know that, by definition, the graph of this relationships will always pass through the origin):

The graph of the Proportional relationship is