Given the function:
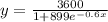
Let's solve for the following:
(a) Graph the function for 0≤x≤15.
This is an exponential function.
This graph will stop increasing at y = 3600
Thus, we have the graph below:
The graph that correctly represents this situation is graph C.
(b) How many students had the virus when it was first discovered?
Here, we are to find the y-intercept.
At the y-intercept, the value of x is zero.
Now, substitute 0 for x and solve for y:
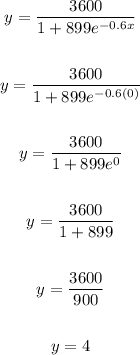
Therefore, the number of students that had the virus when it was first discovered is 4.
(c) What is the upper limit of the number infected by the virus during this period?
Here, we have the limit 0≤x≤15.
This means the upper limit infected during this period will be at x = 15 .
Substitute 15 for x and solve:
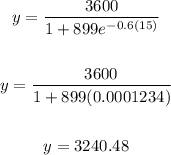
Therefore, the upper limit of the number infected by this virus in [0, 15] is 3240.48
ANSWER:
(b) 4 students
(c) 3240.48