Question

A contractor is pouring a concrete wall is that is four feet high , 18 inches thick and 375 feet long . How many cubic yards of concrete will it take to pour wall ?

Answer 1

We are given the following dimensions of a concrete wall.

Height = 4 feet

Width = 18 inches

Length = 375 feet

We are asked to determine how many cubic yards of concrete will it take to pour the wall.

Recall that the volume of a rectangular prism is given by

`V=l\cdot w\cdot h`

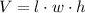
Where l is the length, w is the width, and h is the height.

First, we need to convert all the given dimensions to yards.

There are 3 feet in 1 yard.

`\begin{gathered} h=4*(1)/(3)=(4)/(3)\; yd \\ l=375*(1)/(3)=125\; yd \end{gathered}`

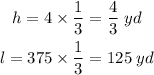
There are 36 inches in 1 yard.

`w=18*(1)/(36)=0.5\; yd`

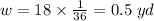
Now, we have all the dimensions in yards, let us substitute them into the formula

`\begin{gathered} V=l\cdot w\cdot h \\ V=125\cdot0.5\cdot(4)/(3) \\ V=83.33\; yd^3 \end{gathered}`

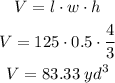
Therefore, 83.33 cubic yards of concrete is required to pour the wall.