Given data:
Initial speed;

Height;
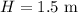
The time of flight for horizontal projectile i s given as,
![T=\sqrt[]{(2H)/(g)}](https://img.qammunity.org/2023/formulas/physics/college/symzfmk6t069tfvvhrniyuwtykgxxmaq6w.png)
Here, g is the acceleration due to gravity.
Substituting all known values,
![\begin{gathered} T=\sqrt[]{\frac{2*1.5\text{ m}}{9.8m/s^2}} \\ =0.55\text{ s} \end{gathered}](https://img.qammunity.org/2023/formulas/physics/college/2n0ijmickx049hl41mdcoeruuux37w5rd6.png)
Therefore, the ball will take 0.55 s to land. Hence, option (a) is the correct choice.
The horizontal range is given as,
![R=u\sqrt[]{(2H)/(g)}](https://img.qammunity.org/2023/formulas/physics/college/zhpsjzqzvxwn967293bnucvr77wr9e3p65.png)
Substituting all known values,
![\begin{gathered} R=(15\text{ m/s})*\sqrt[]{\frac{2*1.5\text{ m}}{9.8\text{ m/s}^2}} \\ =8.29\text{ m} \\ \approx8.3\text{ m} \end{gathered}](https://img.qammunity.org/2023/formulas/physics/college/cok4kj95kcyjkon58eo0bf869820992io6.png)
Therefore, the ball will cover a distance of 8.3 m. Hence, option (c) is the correct choice.