It is given that speech can be 15 minutes, or within that time by 4 minutes.
This means that the speech time can vary around 15 minutes by 4 more or less minutes.
So the limits of speech times are:
Upper limit = 15 + 4 = 19 minutes
Lower limit = 15 - 4 = 11 minutes
So, to find out which absolute value equation represents these limits, we have to solve each absolute value equation.
1st equation:
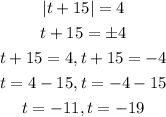
As you can see, these are not the limits we want since they are negative.
So 1st equation is incorrect.
2nd equation:
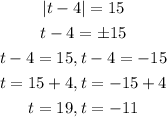
As you can see, these are not limits we want since 11 is negative.
So 2nd equation is also incorrect.
3rd equation:
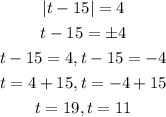
Finally, we got the exact same limits that we wanted t = 19 minutes and t = 11 minutes
Therefore, the correct absolute value equation is the 3rd equation.