Remember that on a normal distribution, 95% of the cases fall between an interval of radius 2 times the standard deviation around the mean. This is, 95% of the cases are inside the interval:
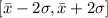
If the mean is equal to 1.4 and the standard deviation is 1.2, then:
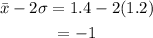
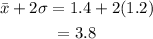
Observe that it is not possible for a student to have less than 0 siblings. Then, the cases in the interval [-1,0) are not real-life cases.
Mathematically, the answer would be:

Which is the interval of 95% confidence for a normal distribution with mean 1.4 and standard deviation of 1.2