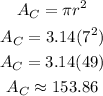To obtain the area that is not sprinkled, subtract the area of the circle from the area of the square field.
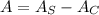
Solve for the area of the square. Substitute the value of the sides into the equation.

Solve for the area of the circle. Substitute the value of the radius into the equation. Note that the measure of the radius is half of the measure of the diameter. Since the diameter is 14 meters, the radius is 7 meters.