Question

The values of x and y vary directly, and when x=48, y=36. Find the value of x when y=18.

Answer 1

When two variables vary directly they follow the next:

`y=k\cdot x`

k is a constant.

Use the given data: when x=48, y=36 o find the value of k:

`\begin{gathered} 36=k\cdot48 \\ \\ (36)/(48)=k \\ \\ k=(3)/(4) \end{gathered}`

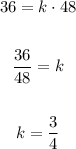
Then, x and y vary directly following the next equation:

`y=(3)/(4)x`

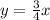
Use the equation above to find x when y=18:

`\begin{gathered} 18=(3)/(4)x \\ \\ 18((4)/(3))=x \\ \\ x=(18\cdot4)/(3) \\ \\ x=(72)/(3) \\ \\ x=24 \end{gathered}`Then, the value of x when y=18 is x=24